Josephson Effect tunneling in superconductors

08/06/1962View on timeline

Josephson effect, flow of electric current between two pieces of superconducting material separated by a thin layer of insulating material. Superconductors are materials that lose all electrical resistance when cooled below a certain temperature near absolute zero. The English physicist Brian D. Josephson predicted the flow of current in 1962 on the basis of the BCS theory (q.v.) of superconductivity. The subsequent experimental verification of the Josephson effect lent support to the BCS theory.

The Josephson current flows only if no battery is connected across the two superconductors. If a battery is inserted, the current oscillates very rapidly so that no net current flows. The presence of magnetic fields near the superconductors influences the Josephson effect, allowing it to be used to measure very weak magnetic fields.

According to the BCS theory, superconductivity is a result of the correlated motion of electrons in the superconducting solid. Part of this correlation is the formation of pairs of electrons called Cooper pairs. According to Josephson, under certain circumstances these Cooper pairs move from one superconductor to the other across the thin insulating layer. Such motion of pairs of electrons constitutes the Josephson current, and the process by which the pairs cross the insulating layer is called Josephson tunneling.

The Josephson effect is central to the operation of the superconducting quantum interference device (SQUID), which is a very sensitive detector of magnetic fields. It is used to measure tiny variations in the magnetic field of the Earth and also of the human body.

Josephson Tunnelling

If two superconducting regions are kept totally isolated from each other the phases of the electron-pairs in the two regions will be unrelated. If the two regions are brought together then as they come close electron-pairs will be able to tunnel across the gap and the two electron-pair waves will become coupled. As the separation decreases the strength of the coupling increases. The tunnelling of the electron-pairs across the gap carries with it a superconducting current as predicted by B.D. Josephson and is called "Josephson Tunnelling'' with the junction between the two superconductors called a "Josephson Junction''.

Like a superconductor this gap has a critical current. If a supercurrent, i_s, flows across a gap between regions with a phase difference, ∆Φ, it is related to the critical current, i_c, by

i_s = i_csin∆Φ

so that the maximum current flows across the gap when there is a phase difference of π/2, where i_s = i_c.

The Josephson Tunnelling Junction is a special case of a more general type of weak link between two superconductors. Other forms include constrictions and point contacts but the general form is of a region between two superconductors which has a much lower critical current and through which a magnetic field can penetrate.

There were a few experiments which can further confirm that their result is indeed what Josephson predicted in his paper:

The effect should be very sensitive to magnetic fields. They did their experiment by blocking external magnetic fields with the aid of a mu-metal shielding, where the measured interior magnetic field is about 6x10^-3 gauss.
The observation of this tunneling occurs only when both metals, the lead and the tin, become superconductors. As pointed out in the very beginning, the Josephson effect is a new kind of tunneling for superconductors (or more precisely, for a junction with two superconductors); therefore one would expect that no Josephson tunneling can be measured before the metals transit into the super-conducting states.
They tried to continue increasing the current through the barrier, in order to burn out the junction, and found that when the junction was being burned out, the characteristic curve changed drastically at the same time. Again, this can be understood by the fact that the SIS junctions are necessary to observe the Josephson effect.

Based on all the above arguments, Anderson and Rowell then made sure that they successfully observed the Josephson tunneling effect. What might concern them was that their measured tunneling current is about one order of magnitude less then the theoretical prediction.

Josephson currents

If two superconductors are separated by an insulating film that forms a low-resistance junction between them, it is found that Cooper pairs can tunnel from one side of the junction to the other. (This process occurs in addition to the single-particle tunneling already described.) Thus, a flow of electrons, called the Josephson current, is generated and is intimately related to the phases of the coherent quantum mechanical wave function for all the superconducting electrons on the two sides of the junction. It was predicted that several novel phenomena should be observable, and experiments have demonstrated them. These are collectively called the Josephson effect or effects.

The first of these phenomena is the passage of current through the junction in the absence of a voltage across the junction. The maximum current that can flow at zero voltage depends on the magnetic flux (Φ) passing through the junction as a result of the magnetic field generated by currents in the junction and elsewhere. The dependence of the maximum zero-voltage current on the magnetic field applied to a junction between two superconductors is shown in figure.

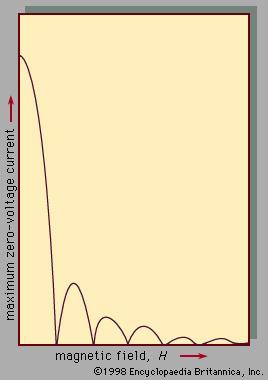
Maximum zero-voltage (Josephson) current passing through a junction by Cooper-pair tunneling as a fu...

A second type of Josephson effect is an oscillating current resulting from a relation between the voltage across the junction and the frequency (ν) of the currents associated with Cooper pairs passing through the junction. The frequency (ν) of this Josephson current is given by ν = 2eV/h, where e is the charge of the electron. Thus, the frequency increases by 4.84 × 10¹⁴ hertz (cycles per second) for each additional volt applied to the junction. This effect can be demonstrated in various ways. The voltage can be established with a source of direct-current (DC) power, for instance, and the oscillating current can be detected by the electromagnetic radiation of frequency (ν) that it generates. Another method is to expose the junction to radiation of another frequency (ν′) generated externally. It is found that a graph of the DC current versus voltage has current steps at values of the voltage corresponding to Josephson frequencies that are integral multiples (n) of the external frequency (ν = nν′); that is, V = nhν′/2e. The observation of current steps of this type has made it possible to measure h/e with far greater precision than by any other method and has therefore contributed to a knowledge of the fundamental constants of nature.

The Josephson effect has been used in the invention of novel devices for extremely high- sensitivity measurements of currents, voltages, and magnetic fields.

Josephson junction

A Josephson junction is made by sandwiching a thin layer of a nonsuperconducting material between two layers of superconducting material.

To understand the unique and important features of Josephson junctions, it's first necessary to understand the basic concepts and features of superconductivity. If you cool many metals and alloys to very low temperatures (within 20 degrees or less of absolute zero), a phase transition occurs. At this "critical temperature," the metal goes from what is known as the normal state, where it has electrical resistance, to the superconducting state, where there is essentially no resistance to the flow of direct electrical current. The newer high-temperature superconductors, which are made from ceramic materials, exhibit the same behavior but at warmer temperatures.

What occurs is that the electrons in the metal become paired. Above the critical temperature, the net interaction between two electrons is repulsive. Below the critical temperature, though, the overall interaction between two electrons becomes very slightly attractive, a result of the electrons' interaction with the ionic lattice of the metal.

This very slight attraction allows them to drop into a lower energy state, opening up an energy "gap." Because of the energy gap and the lower energy state, electrons can move (and therefore current can flow) without being scattered by the ions of the lattice. When the ions scatter electrons, it causes electrical resistance in metals. There is no electrical resistance in a superconductor, and therefore no energy loss. There is, however, a maximum supercurrent that can flow, called the critical current. Above this critical current the material is normal. There is one other very important property: when a metal goes into the superconducting state, it expels all magnetic fields, as long as the magnetic fields are not too large.

In a Josephson junction, the nonsuperconducting barrier separating the two superconductors must be very thin. If the barrier is an insulator, it has to be on the order of 30 angstroms thick or less. If the barrier is another metal (nonsuperconducting), it can be as much as several microns thick. Until a critical current is reached, a supercurrent can flow across the barrier; electron pairs can tunnel across the barrier without any resistance. But when the critical current is exceeded, another voltage will develop across the junction. That voltage will depend on time--that is, it is an AC voltage. This in turn causes a lowering of the junction's critical current, causing even more normal current to flow and a larger AC voltage.

The frequency of this AC voltage is nearly 500 gigahertz (GHz) per millivolt across the junction. So, as long as the current through the junction is less than the critical current, the voltage is zero. As soon as the current exceeds the critical current, the voltage is not zero but oscillates in time. Detecting and measuring the change from one state to the other is at the heart of the many applications for Josephson junctions.

Electronic circuits can be built from Josephson junctions, especially digital logic circuitry. Many researchers are working on building ultrafast computers using Josephson logic. Josephson junctions can also be fashioned into circuits called SQUIDs--an acronym for superconducting quantum interference device. These devices are extremely sensitive and very useful in constructing extremely sensitive magnetometers and voltmeters. For example, one can make a voltmeter that can measure picovolts. That's about 1,000 times more sensitive than other available voltmeters.

A SQUID consists of a loop with two Josephson junctions interrupting the loop. A SQUID is extremely sensitive to the total amount of magnetic field that penetrates the area of the loop--the voltage that you measure across the device is very strongly correlated to the total magnetic field around the loop.

SQUIDs are being used for research in a variety of areas. Since the brain operates electrically, one can, by sensing the magnetic fields created by neurological currents, monitor the activity of the brain--or the heart. You can also use a SQUID magnetometer for geological research, detecting remnants of past geophysical changes of the earth's field in rocks. Similarly, changes in the ambient magnetic field are created by submarines passing below the surface of the ocean, and the U.S. Navy is very interested in SQUIDs for submarine detection. SQUIDs are also of considerable use in the research laboratory in specially designed voltmeters, in magnetometers and susceptometers and in scanning SQUID microscopes. In this last instrument, a SQUID is scanned across the surface of a sample, and changes in magnetism at the surface of the sample produce an image.

POSSIBLE NEW EFFECTS IN SUPERCONDUCTIVE TUNNELLING