Hall Effect

Hall effect, development of a transverse electric field in a solid material when it carries an electric current and is placed in a magnetic field that is perpendicular to the current. This phenomenon was discovered in 1879 by the U.S. physicist Edwin Herbert Hall.

The electric field, or Hall field, is a result of the force that the magnetic field exerts on the moving positive or negative particles that constitute the electric current. Whether the current is a movement of positive particles, negative particles in the opposite direction, or a mixture of the two, a perpendicular magnetic field displaces the moving electric charges in the same direction sideways at right angles to both the magnetic field and the direction of current flow. The accumulation of charge on one side of the conductor leaves the other side oppositely charged and produces a difference of potential. An appropriate meter may detect this difference as a positive or negative voltage. The sign of this Hall voltage determines whether positive or negative charges are carrying the current.

In metals, the Hall voltages are generally negative, indicating that the electric current is composed of moving negative charges, or electrons. The Hall voltage is positive, however, for a few metals such as beryllium, zinc, and cadmium, indicating that these metals conduct electric currents by the movement of positively charged carriers called holes. In semiconductors, in which the current consists of a movement of positive holes in one direction and electrons in the opposite direction, the sign of the Hall voltage shows which type of charge carrier predominates. The Hall effect can be used also to measure the density of current carriers, their freedom of movement, or mobility, as well as to detect the presence of a current on a magnetic field.

The Hall voltage that develops across a conductor is directly proportional to the current, to the magnetic field, and to the nature of the particular conducting material itself; the Hall voltage is inversely proportional to the thickness of the material in the direction of the magnetic field. Because various materials have different Hall coefficients, they develop different Hall voltages under the same conditions of size, electric current, and magnetic field. Hall coefficients may be determined experimentally and may vary with temperature.

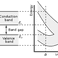
The Hall effect is due to the nature of the current in a conductor. Current consists of the movement of many small charge carriers, typically electrons, holes, ions or all three. When a magnetic field is present, these charges experience a force, called the Lorentz force. When such a magnetic field is absent, the charges follow approximately straight, 'line of sight' paths between collisions with impurities, phonons, etc. However, when a magnetic field with a perpendicular component is applied, their paths between collisions are curved, thus moving charges accumulate on one face of the material. This leaves equal and opposite charges exposed on the other face, where there is a scarcity of mobile charges. The result is an asymmetric distribution of charge density across the Hall element, arising from a force that is perpendicular to both the 'line of sight' path and the applied magnetic field. The separation of charge establishes an electric field that opposes the migration of further charge, so a steady electric potential is established for as long as the charge is flowing.

In classical electromagnetism electrons move in the opposite direction of the current I (by convention "current" describes a theoretical "hole flow"). In some semiconductors it appears "holes" are actually flowing because the direction of the voltage is opposite to the derivation below.

For a simple metal where there is only one type of charge carrier (electrons), the Hall voltage V_H can be derived by using the Lorentz force and seeing that, in the steady-state condition, charges are not moving in the y-axis direction. Thus, the magnetic force on each electron in the y-axis direction is cancelled by a y-axis electrical force due to the buildup of charges.

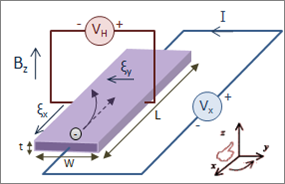
At some distance from the current-introducing contacts, electrons pile up on the left side and deplete from the right side, which creates an electric field ξ_y in the direction of the assigned V_H. V_H is negative for some semiconductors where "holes" appear to flow. In steady-state, ξ_y will be strong enough to exactly cancel out the magnetic force, thus the electrons follow the straight arrow (dashed).

The v_x term is the drift velocity of the current which is assumed at this point to be holes by convention. The v_xB_z term is negative in the y-axis direction by the right hand rule.

F = q(E + v × B)

In steady state, F = 0, so 0 = E_y − v_xB_z, where E_y is assigned in the direction of the y-axis, (and not with the arrow of the induced electric field ξ_y as in the image (pointing in the −y direction), which tells you where the field caused by the electrons is pointing). In wires, electrons instead of holes are flowing, so v_x → −v_x and q → −q. Also E_y = −V_H/w. Substituting these changes gives

V_H = v_xB_zw

The conventional "hole" current is in the negative direction of the electron current and the negative of the electrical charge which gives I_x = ntw(−v_x)(−e) where n is charge carrier density, tw is the cross-sectional area, and −e is the charge of each electron. Solving for w and plugging into the above gives the Hall voltage:

V_H = I_xB_z/nte

If the charge build up had been positive (as it appears in some semiconductors), then the V_H assigned in the image would have been negative (positive charge would have built up on the left side). The Hall coefficient is defined as

R_H = E_y/j_xB_z

where j is the current density of the carrier electrons, and E_y is the induced electric field. In SI units, this becomes

R_H = E_y/jxB = V_Ht/IB = -1/ne

(The units of RH are usually expressed as m³/C, or Ω·cm/G, or other variants.) As a result, the Hall effect is very useful as a means to measure either the carrier density or the magnetic field.

One very important feature of the Hall effect is that it differentiates between positive charges moving in one direction and negative charges moving in the opposite. The Hall effect offered the first real proof that electric currents in metals are carried by moving electrons, not by protons. The Hall effect also showed that in some substances (especially p-type semiconductors), it is more appropriate to think of the current as positive "holes" moving rather than negative electrons. A common source of confusion with the Hall effect is that holes moving to the left are really electrons moving to the right, so one expects the same sign of the Hall coefficient for both electrons and holes. This confusion, however, can only be resolved by modern quantum mechanical theory of transport in solids.

The sample inhomogeneity might result in spurious sign of the Hall effect, even in ideal van der Pauw configuration of electrodes. For example, positive Hall effect was observed in evidently n-type semiconductors. Another source of artifact, in uniform materials, occurs when the sample's aspect ratio is not long enough: the full Hall voltage only develops far away from the current-introducing contacts, since at the contacts the transverse voltage is shorted out to zero.

Hall Probe

REFERENCES

Encyclopaedia Britannica. Available in: https://www.britannica.com/science/Hall-effect. Access in: 07/10/2018.

Georgia State University. Available in: http://hyperphysics.phy-astr.gsu.edu/hbase/magnetic/Hall.html. Access in: 07/10/2018.

Christian-Albrechts-Universität zu Kiel. Available in: https://www.tf.uni-kiel.de/matwis/amat/mw2_ge/kap_2/backbone/r2_1_3.html. Access in: 07/10/2018.

Wikipedia. Available in: https://en.wikipedia.org/wiki/Hall_effect Access in: 07/10/2018.