Schrödinger's Quantum Mechanics

01/01/1926View on timeline

Erwin Schrödinger, Austrian theoretical physicist who contributed to the wave theory of matter and to other fundamentals of quantum mechanics. In 1926 Schroedinger sought to find a wave equation for the matter waves of de Broglie. The fact that there are only certain states or permissible energies that an electron can have on an atom is similar to a standing wave.

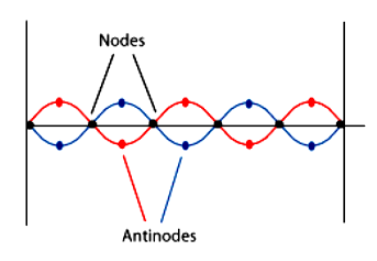
Note that there are points where the displacement is zero, or nodes, which occurs along the standing wave. Nodes are marked with black dots. Since the string is attached at the ends, this generates a limitation that only certain wavelengths are allowed for a standing wave. As such, the vibrations are quantized.

We can think of electrons as stationary waves of matter that have certain permissible energies. Schrödinger formulated a model of the atom which considered that electrons could be treated as waves of matter. No known physical quantity explains the nature of these waves, so the Greek letter Ψ was used to designate the wave function of matter. In this way, Schrödinger determines that the matter wave of a free particle, that is, the electron, is properly the light like a single ray of light if "propagating". However, formulating an equation of waves of matter is necessary to know how to interpret it. The problem of interpretation is linked to the conditions that must be imposed on the solutions of the equation to be physically acceptable, in particular the boundary conditions that must be satisfied.

In quantum mechanics, the Schrödinger equation is a mathematical equation that describes the changes over time of a physical system in which quantum effects, such as wave–particle duality, are significant. These systems are referred to as quantum (mechanical) systems. The equation is considered a central result in the study of quantum systems, and its derivation was a significant landmark in the development of the theory of quantum mechanics.

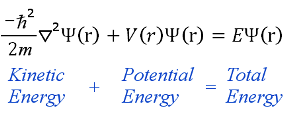
Schrödinger's time-independent equation.

The solutions to Schrödinger’s equation, unlike the solutions to Newton’s equations, are wave functions that can only be related to the probable occurrence of physical events. The definite and readily visualized sequence of events of the planetary orbits of Newton is, in quantum mechanics, replaced by the more abstract notion of probability.

This aspect of the quantum theory made Schrödinger and several other physicists profoundly unhappy, and he devoted much of his later life to formulating philosophical objections to the generally accepted interpretation of the theory that he had done so much to create. His most famous objection was the 1935 thought experiment that later became known as Schrödinger’s cat. A cat is locked in a steel box with a small amount of a radioactive substance such that after one hour there is an equal probability of one atom either decaying or not decaying. If the atom decays, a device smashes a vial of poisonous gas, killing the cat. However, until the box is opened and the atom’s wave function collapses, the atom’s wave function is in a superposition of two states: decay and non-decay. Thus, the cat is in a superposition of two states: alive and dead. Schrödinger thought this outcome “quite ridiculous,” and when and how the fate of the cat is determined has been a subject of much debate among physicists.

In the Copenhagen interpretation of quantum mechanics, the wave function is the most complete description that can be given of a physical system. Solutions to Schrödinger's equation describe not only molecular, atomic, and subatomic systems, but also macroscopic systems, possibly even the whole universe. Schrödinger's equation is central to all applications of quantum mechanics including quantum field theory which combines special relativity with quantum mechanics. Theories of quantum gravity, such as string theory, also do not modify Schrödinger's equation.

The Schrödinger equation is not the only way to study quantum mechanical systems and make predictions, as there are other quantum mechanical formulations such as matrix mechanics, introduced by Werner Heisenberg, and path integral formulation, developed chiefly by Richard Feynman. Paul Dirac incorporated matrix mechanics and the Schrödinger equation into a single formulation.

Schrödinger's paper in English

Definition of the Schrödinger Equation