Dark Matter
Dark matter is one of the great unsolved mysteries of modern astronomy. We've reached the point where we know most matter in the cosmos is made of matter that interacts weakly with light if at all, but drives much of the gravitational interactions between galaxies. While it's often portrayed as a modern idea added simply to shoehorn observations into the standard model, it actually has a history spanning more than a century, and the theory of dark matter has been refined and improved as we've learned more about our Universe.
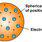
Dark matter is a hypothetical form of matter that is thought to account for approximately 85% of the matter in the universe, and about a quarter of its total energy density. The majority of dark matter is thought to be non-baryonic in nature, possibly being composed of some as-yet undiscovered subatomic particles. Its presence is implied in a variety of astrophysical observations, including gravitational effects that cannot be explained unless more matter is present than can be seen. For this reason, most experts think dark matter to be ubiquitous in the universe and to have had a strong influence on its structure and evolution. The name dark matter refers to the fact that it does not appear to interact with observable electromagnetic radiation, such as light, and is thus invisible (or 'dark') to the entire electromagnetic spectrum, making it extremely difficult to detect using usual astronomical equipment
The origins of dark matter can be traced to the 1600s. Soon after Isaac Newton presented his theory of universal gravity, some astronomers began to speculate about the existence of objects that might emit little or no light, but could still be known by their gravitational tug on bright objects like stars and planets. This idea was strengthened in the 1700s when Pierre Laplace argued that some objects might be massive enough to trap any light they emit (a simplistic idea of a black hole), and by the 1800s Urbain Le Verrier and John Couch Adams used gravitational anomalies in the motion of Uranus to predict the presence of Neptune. By this point astronomers had demonstrated the presence of dark nebulae, seen only by the light they absorb from bright objects behind them. It was clear that there was more in the Universe than could be seen by visible light.
Our modern take on dark matter as a major contributor to galactic mass can be traced to Fritz Zwicky. In 1933 he studied the motion of galaxies within the Coma Cluster. The Coma Cluster is a galactic supercluster containing more than 1,000 galaxies. Since these galaxies are gravitationally bound, the speed of these galaxies can provide a measure of the cluster's mass. Basically, the more mass the cluster has, the wider the distribution of galactic speeds following a relation known as the virial theorem. A few years earlier Edwin Hubble had estimated that the Coma Cluster contained about 800 galaxies, each containing about a billion stars. Using the virial theorem Zwicky calculated a cluster mass more than 500 times larger than that of Hubble. Zwicky noted that if his measurements held true "dark matter is present in much greater amount than luminous matter."
Over the next couple decades the virial theorem was applied to other galaxy clusters with similar results. Not everyone accepted these results, largely because the virial theorem is a statistical calculation that depends upon certain assumptions. For example, it assumes the clusters are gravitationally bound. Perhaps the galaxies in these clusters are actually flying away from each other, so that the virial theorem simply doesn't apply. But there was another line of evidence to support dark matter. One that couldn't be so easily dismissed.

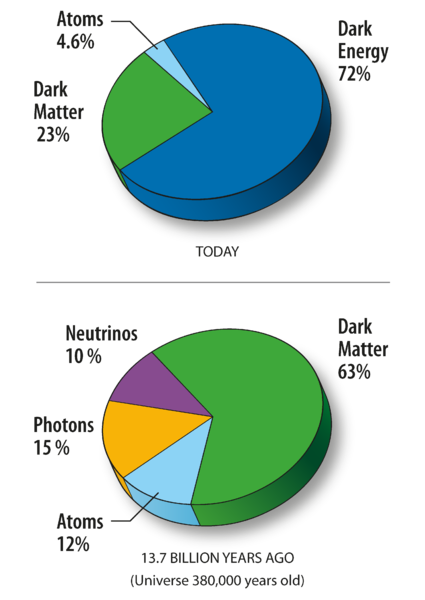
The primary evidence for dark matter is that calculations show that many galaxies would fly apart instead of rotating, or would not have formed or move as they do, if they did not contain a large amount of unseen matter. Other lines of evidence include observations in gravitational lensing, from the cosmic microwave background, from astronomical observations of the observable universe's current structure, from the formation and evolution of galaxies, from mass location during galactic collisions, and from the motion of galaxies within galaxy clusters. In the standard Lambda-CDM model of cosmology, the total mass–energy of the universe contains 5% ordinary matter and energy, 27% dark matter and 68% of an unknown form of energy known as dark energy. Thus, dark matter constitutes 85% of total mass, while dark energy plus dark matter constitute 95% of total mass–energy content.
Because dark matter has not yet been observed directly, it must barely interact with ordinary baryonic matter and radiation. The primary candidate for dark matter is some new kind of elementary particle that has not yet been discovered, in particular, weakly-interacting massive particles (WIMPs), or gravitationally-interacting massive particles (GIMPs). Many experiments to directly detect and study dark matter particles are being actively undertaken, but none has yet succeeded. Dark matter is classified as cold, warm, or hot according to its velocity (more precisely, its free streaming length). Current models favor a cold dark matter scenario, in which structures emerge by gradual accumulation of particles.
Although the existence of dark matter is generally accepted by the scientific community, some astrophysicists, intrigued by certain observations that do not fit the dark matter theory, argue for various modifications of the standard laws of general relativity, such as modified Newtonian dynamics, tensor–vector–scalar gravity, or entropic gravity. These models attempt to account for all observations without invoking supplemental non-baryonic matter.
In the early 1900s astronomers began to look at the spectra of galaxies. From this they could determine the speeds of stars as a function of their distance from galactic center, known as a galactic rotation curve. Seen in visible light, most galaxies have a bright center, dimming as you move away from the center. This would imply most of the stars (and thus most of the mass) is located near the center of a galaxy. If that's the case, one would expect stars far from the center to move much more slowly than stars near the center, just as in our solar system Earth orbits the Sun much more quickly than distant Pluto. When Max Wolf and Vesto Slipher measured the rotation curve of the Andromeda galaxy, they found it was basically flat, meaning that stars moved at the same speed regardless of their distance from galactic center.
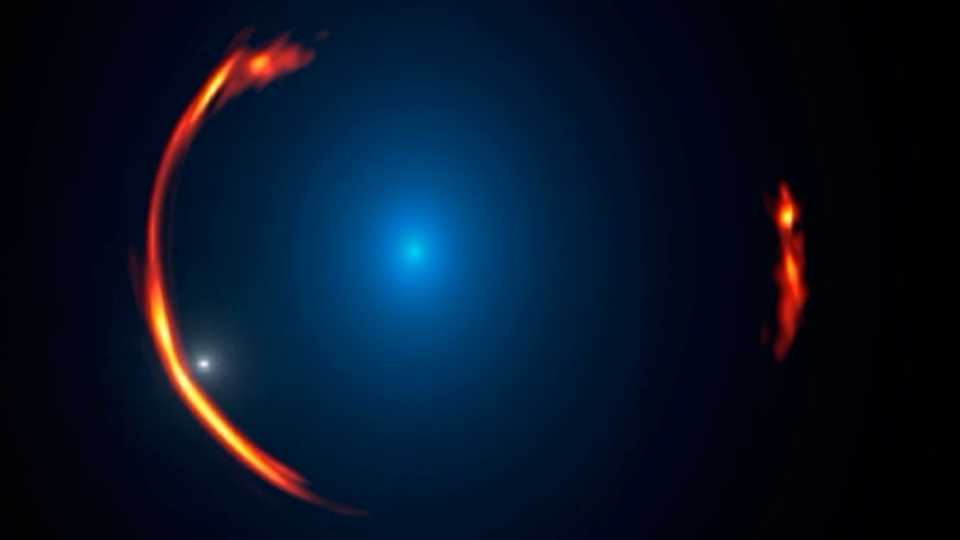
One solution to this mystery was that Andromeda is surrounded by a halo of dark matter so that its mass is not concentrated in the center. While other galaxies showed similar rotation curves, seeming to support the presence of dark matter, even Fritz Zwicky was skeptical. Gas and dust within a galaxy might exert some kind of drag on fast moving stars, he argued, thus flattening the rotation curves. But by the 1950s radio astronomy had progressed to the point where it could detect monatomic hydrogen through the famous 21 centimeter line. Radio observations of both the Andromeda galaxy and our own Milky Way galaxy showed similarly flat rotation curves. Since hydrogen is by far the most abundant element in the Universe, the results proved that not only stars, but the gas of any dark nebulae were orbiting the galaxies at similar speeds. Either galaxies contained significant dark matter, or our understanding of gravity was very wrong.
As the evidence for dark matter grew, it soon became clear that there was a serious problem. Assuming our gravitational theories were correct, dark matter must be far more plentiful than luminous matter both in galaxies and among galactic clusters. If this dark matter consisted of things like dark nebulae, their presence should be detectable by the light they absorb. If so much dark matter exists, it must not only be non-luminous, it must not absorb light either. It couldn't simply be regular matter that is cold and dark, but must be something very different. This was such a radial idea that many astronomers questioned the validity of Newtonian gravity. By the 1980s several alternative gravitational models, the most famous of which was Modified Newtonian Dynamics (MoND), proposed by Mordehai Milgrom. While these models did work well for things like dwarf galaxies, they worked horribly with things like galactic clusters. Dark matter models were not without their problems, but they agreed more readily with observations.

In the past couple decades data gathered from gravitational lensing and deep sky surveys have allowed us to further refine our dark matter models. From the large scale distribution of galaxies we know that dark matter must be cold and slow moving, so the countless neutrinos that zip through the cosmos at nearly the speed of light cannot account for dark matter. From gravitational lensing we know the distribution of dark matter within galaxies. By observing the distribution of dark matter within colliding galaxies we know that not only does dark matter not interact with light, it also doesn't interact strongly with regular matter or itself. While this further verifies the existence of dark matter, it also makes it more difficult to determine just what dark matter is.
The most recent challenge for dark matter has been to determine its composition. The most popular idea is that they are Weakly Interacting Massive Particles (WIMPs), but these particles should be detectible by the same experiments used to observe astrophysical neutrinos. So far, no evidence for these particles has been forthcoming. Direct efforts to detect dark matter have only served to eliminate our options for dark matter. After studying dark matter for more than a century, it continues to elude us.
Observational evidence
Galaxy rotation curves
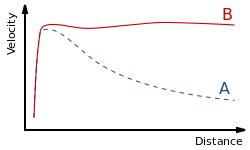
The arms of spiral galaxies rotate around the galactic center. The luminous mass density of a spiral galaxy decreases as one goes from the center to the outskirts. If luminous mass were all the matter, then we can model the galaxy as a point mass in the centre and test masses orbiting around it, similar to the Solar System. From Kepler's Second Law, it is expected that the rotation velocities will decrease with distance from the center, similar to the Solar System. This is not observed. Instead, the galaxy rotation curve remains flat as distance from the center increases.
If Kepler's laws are correct, then the obvious way to resolve this discrepancy is to conclude that the mass distribution in spiral galaxies is not similar to that of the Solar System. In particular, there is a lot of non-luminous matter (dark matter) in the outskirts of the galaxy.
Velocity dispersions
Stars in bound systems must obey the virial theorem. The theorem, together with the measured velocity distribution, can be used to measure the mass distribution in a bound system, such as elliptical galaxies or globular clusters. With some exceptions, velocity dispersion estimates of elliptical galaxies do not match the predicted velocity dispersion from the observed mass distribution, even assuming complicated distributions of stellar orbits.
As with galaxy rotation curves, the obvious way to resolve the discrepancy is to postulate the existence of non-luminous matter.
Galaxy clusters
Galaxy clusters are particularly important for dark matter studies since their masses can be estimated in three independent ways:
- From the scatter in radial velocities of the galaxies within clusters;
- From X-rays emitted by hot gas in the clusters. From the X-ray energy spectrum and flux, the gas temperature and density can be estimated, hence giving the pressure; assuming pressure and gravity balance determines the cluster's mass profile;
- Gravitational lensing (usually of more distant galaxies) can measure cluster masses without relying on observations of dynamics (e.g., velocity).
Generally, these three methods are in reasonable agreement that dark matter outweighs visible matter by approximately 5 to 1
Gravitational lensing
One of the consequences of general relativity is that massive objects (such as a cluster of galaxies) lying between a more distant source (such as a quasar) and an observer should act as a lens to bend the light from this source. The more massive an object, the more lensing is observed.
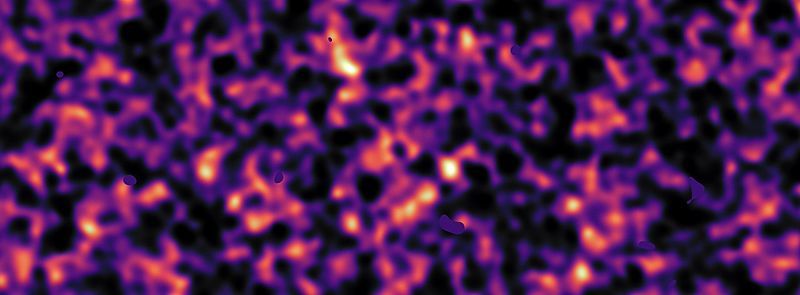
Strong lensing is the observed distortion of background galaxies into arcs when their light passes through such a gravitational lens. It has been observed around many distant clusters including Abell 1689. By measuring the distortion geometry, the mass of the intervening cluster can be obtained. In the dozens of cases where this has been done, the mass-to-light ratios obtained correspond to the dynamical dark matter measurements of clusters. Lensing can lead to multiple copies of an image. By analyzing the distribution of multiple image copies, scientists have been able to deduce and map the distribution of dark matter around the MACS J0416.1-2403 galaxy cluster.
Weak gravitational lensing investigates minute distortions of galaxies, using statistical analyses from vast galaxy surveys. By examining the apparent shear deformation of the adjacent background galaxies, the mean distribution of dark matter can be characterized. The mass-to-light ratios correspond to dark matter densities predicted by other large-scale structure measurements. Dark matter does not bend light itself; mass (in this case the mass of the dark matter) bends spacetime. Light follows the curvature of spacetime, resulting in the lensing effect.
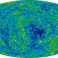
Cosmic microwave background
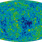
Although both dark matter and ordinary matter are matter, they do not behave in the same way. In particular, in the early universe, ordinary matter was ionized and interacted strongly with radiation via Thomson scattering. Dark matter does not interact directly with radiation, but it does affect the CMB by its gravitational potential (mainly on large scales), and by its effects on the density and velocity of ordinary matter. Ordinary and dark matter perturbations, therefore, evolve differently with time and leave different imprints on the cosmic microwave background (CMB).
The cosmic microwave background is very close to a perfect blackbody but contains very small temperature anisotropies of a few parts in 100,000. A sky map of anisotropies can be decomposed into an angular power spectrum, which is observed to contain a series of acoustic peaks at near-equal spacing but different heights. The series of peaks can be predicted for any assumed set of cosmological parameters by modern computer codes such as CMBFast and CAMB, and matching theory to data, therefore, constrains cosmological parameters. The first peak mostly shows the density of baryonic matter, while the third peak relates mostly to the density of dark matter, measuring the density of matter and the density of atoms.
The CMB anisotropy was first discovered by COBE in 1992, though this had too coarse resolution to detect the acoustic peaks. After the discovery of the first acoustic peak by the balloon-borne BOOMERanG experiment in 2000, the power spectrum was precisely observed by WMAP in 2003-12, and even more precisely by the Planck spacecraft in 2013-15. The results support the Lambda-CDM model.
The observed CMB angular power spectrum provides powerful evidence in support of dark matter, as its precise structure is well fitted by the Lambda-CDM model, but difficult to reproduce with any competing model such as modified Newtonian dynamics (MOND).




REFERENCES
Forbes. Available in: https://www.forbes.com/sites/briankoberlein/2016/09/19/the-dark-history-of-dark-matter/#6cc77b518946. Access in: 25/11/2018.
Wikipedia. Available in: https://en.wikipedia.org/wiki/Dark_matter. Access in: 25/11/2018.
Cosmos Magazine. Available in: https://web.archive.org/web/20151120074531/http://archive.cosmosmagazine.com/features/what-dark-matter/. Access in: 25/11/2018.
Nasa. Available in: https://www.nasa.gov/home/hqnews/2006/aug/HQ_06297_CHANDRA_Dark_Matter.html. Access in: 25/11/2018.
Encyclopædia Britannica. Available in: https://www.britannica.com/science/dark-matter. Access in: 25/11/2018.
University of Sheffield. Available in: https://www.sheffield.ac.uk/polopoly_fs/1.514231!/file/PHY3262015Topic3Notes.pdf. Access in: 25/11/2018.











































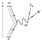
























0 comments
Sign in or create a free account